Antennas, Antenna Cables, Wireless Products: Technical Articles
VSWR: Impedance Matching in Antennas & Antenna Cables
VSWR Explained:
Voltage Standing Wave Ratio (VSWR) is a crucial Indicator of antenna performance (along with gain/dBi): The lower the VSWR, the more efficient the antenna.
- VSWR is a measure of the efficiency of connectors).
- VSWR and return loss are two parameters used to determine whether an antenna is effectively impedance-matched internally and to the antenna cable or connectors (line of signal transmission) it is connected to.
- For a radio receiver or transmitter to deliver power to an antenna, the impedance of the radio and the antenna cable should match well with the impedance of an antenna.
- 50 Ohms is the correct impedance for antennas and antenna cables for all of the following applications: WiFi (802.11AC, N, G, A, B), LTE (4G), GSM (3G), ISM, IoT wireless protocols including Bluetooth, RFID, LoRa, NB-IoT, ZigBee, LTE-m. All of Data Alliance's antennas, antenna cables, and adapters are impedance-matched to 50 Ohms.
- Often, there is a bandwidth range that antennas must satisfy and is defined in terms of VSWR.
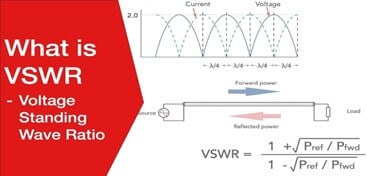
Voltage Standing Wave Ratio (VSWR) measures the efficiency of radio frequency (RF) power transmission from its source through a transmission line to an antenna.
- In a perfect system, 100% of the RF power is transmitted through a "loss-less" line.
- In reality, imperfections in transmission with mismatched impedance cause RF energy to be reflected to the source.
- VSWR expresses this variance between maximum and minimum voltage across the transmission line.
VSWR varies from a ratio of 1, indicating a system with no impedance between components, to an absolute mismatch where VSWR is anything up to ∞. A VSWR value of 2 or under is typically acceptable for antennae in communications systems.
The lower the VSWR, the better the antenna impedance matched to the transmission line and the higher the power delivered. Furthermore, a small VSWR reduces reflections from the antenna. 1.0 is the minimum VSWR where no power is reflected, and it’s the ideal condition for an antenna.
Return loss, measured in dB, which indicates how much incident power is reflected in the source of the signal, works in the opposite way from VSWR. The higher the return loss, the more power to an antenna, meaning you are losing less power and signal strength. Low return loss affects the antenna’s capability to radiate, affecting transmission efficiency. For an antenna to have good signal strength and efficient performance, VSWR should be low while dBi should be high.
VSWR related to Antenna Gain (dBi): The dBi value measures an antenna's gain. Antenna gain is a performance metric for the combined performance of an antenna's electrical efficiency and directivity. Depending on the type of antenna (transmitting vs. receiving), gain describes the conversion capability of an antenna, i.e., to convert electrical power into radio waves or radio waves into electrical energy.
For there to be a maximum power transfer (between the transmitter/receiver, the cable, and the antenna), the transmitter/receiver's load impedance must match the cable's impedance. The cable load impedance also has to match the antenna's impedance. If there is a perfect load match, this will result in antenna gain (perfect signal strength and antenna efficiency). Any load mismatches cause a power transfer loss.
Gain is measured in decibels (dB). The dBi value reflects the antenna's directivity and electrical efficiency, differentiating between transmitting and receiving antennae for enhanced characterization of antenna performance.
Impedance-Matching to 50 Ohms
- Impedance is opposition encountered by electrical energy as it moves away from its source.
- Synchronizing load and source impedance will cancel out the effect, leading to maximum power transfer.
- This is known as the maximum power transfer theorem: Maximum power transfer theorem is critical in radio frequency transmission assemblies, particularly in setting RF antennas.
Impedance matching is critical to the efficient functioning of RF setups where you want to move voltage and power optimally. In RF design, the matching of source and load impedances will maximize the transmission of RF power. Antennas will receive maximum or optimal power transfer where their impedance is matched to the output impedance of the transmission source.
50 Ohm impedance is the standard for designing most RF systems and components. The coaxial cable, which underpins the connectivity in various RF applications, has a typical impedance of 50 Ohms. RF research undertaken in the 1920s found that the optimal impedance for the transfer of RF signals would be between 30 and 60 ohms, depending on voltage and power transfer. Having relatively standardized impedance allows for matching cabling and components such as WiFi or Bluetooth antennas, PCBs, and attenuators. Several key antenna types have an impedance of 50 Ohms, including ZigBee, GSM, GPS, and LoRa.
A mismatch in impedance leads to voltage and current reflections. In RF setups, signal power will be reflected back to its source, the proportion being according to the degree of mismatch. This can be characterized using the Voltage Standing Wave Ratio (VSWR), which measures the efficiency of transferring RF power from its source into a load, such as an antenna.
Mismatching between source and load impedances, such as a 75-Ohm antenna and 50-Ohm coax cabling, can be overcome using a range of impedance matching devices, such as resistors in series, transformers, surface-mounted impedance matching pads, or antenna tuners.
In electronics, impedance matching involves creating or altering a circuit, electronic application, or component set so that the impedance of the electrical load matches the impedance of the power or driving source. The circuit is engineered or geared so that the impedances appear the same.
RF Connectors & Coax Cables for IoT Wireless







